
On May 17, 2023, Wei Ping's research group of the Cell and Genetic Circuit Design Center of the Institute of Synthetic Biology of Shenzhen Institute of Advanced Technology, Chinese Academy of Sciences, and the research group of Morgan H. Jensen, the president of the Royal Danish Academy of Sciences and Letters, jointly published the research paper in Cell Systems under the title of “Coupled oscillator cooperativity as a control mechanism in chronobiology”. They constructed a ternary coupled oscillation system by synthetic biology method, and, based on mathematical models, delved into the regulatory mechanism of multiple coupled oscillation systems in living systems for the first time.

Screenshot of the published paper
Link of the paper: https://doi.org/10.1016/j.cels.2023.04.001
In the physical world, the superposition of multiple oscillatory waves may cause complex phenomena such as interference fringes. In fact, periodic oscillatory dynamic behavior not only occurs in physical phenomena, but also widely exists in living systems. The periodic changes in the components of biological systems, from the biological clock, somite development, and nerve impulses, to the cell cycle, immunoregulation, and signal transduction, help us maintain stable rhythms, generate correct developmental structures, and make precise and quantitative responses to external information; in these processes, interactions between multiple oscillation signals are often involved. What regulatory principles are involved in this complex system is an important but difficult problem.
Interference of same frequency oscillatory waves (The picture comes from the Internet)
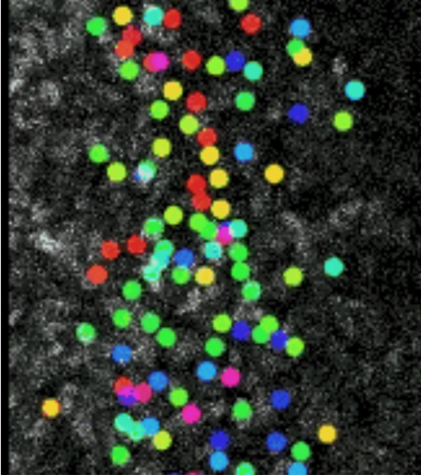
Traveling waves of Hes7 during the somite development of mouse (quoted from Yoshioka-Kobayashi et al., 2020)
Figure 1 Oscillations in physical and biological systems
As early as the 17th century, physicist Huygens discovered that two coupled pendulums could magically synchronize under specific conditions, and research on such problems led to the famous coupled oscillators model in nonlinear dynamics. This model can predict the conditions under which two interrelated (or unidirectionally correlated) oscillators will produce frequency locking, multistable limit cycles and chaos. However, the classical coupled oscillator model only describes the simple case where the number of oscillators n=2, and does not explore the complexity brought by the simultaneous presence of multiple oscillation signals in real biological systems when n≥3. Will multiple oscillators make the system more chaotic or more stable? Is there any new regulatory mechanism for this issue? To explore this issue, it is necessary not only to expand the theoretical model, but also to establish a simple and clear experimental system without interference.

Two metronomes interacted with each other through the bottom board to form an anti-phase synchronization (The picture comes from the Internet)
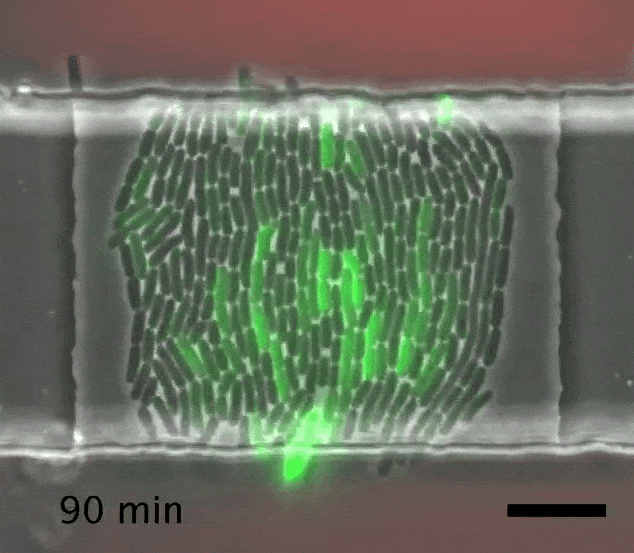
The synthetic oscillatory system in Escherichia coli was synchronized by periodic arabinose stimulation (quoted from Mondragón-Palomino et al., 2011)
Figure 2 Coupled oscillators in mechanical and biological systems
To explore this problem, the author of the paper modified the NF-κB oscillatory system previously artificially constructed in yeast cells (Zhang et al., 2017), further increasing the complexity of the system and enabling it to be controlled by two external signals. The core design of this system is to form a periodic activation process of NF-κB in yeast cells through the grafting of signaling molecules based on the negative feedback circuit composed of NF-κB/IκB signaling molecules related to immune response in human cells. By using the microfluidic chip system, the author could precisely program the oscillation period and intensity of two external input signals, thus achieving a three-oscillation system where 2 external periodic signals interact with 1 internal periodic signal, which provided a living cell experimental system that can be quantitatively regulated for studying the multi-oscillation coupling problem in life processes.
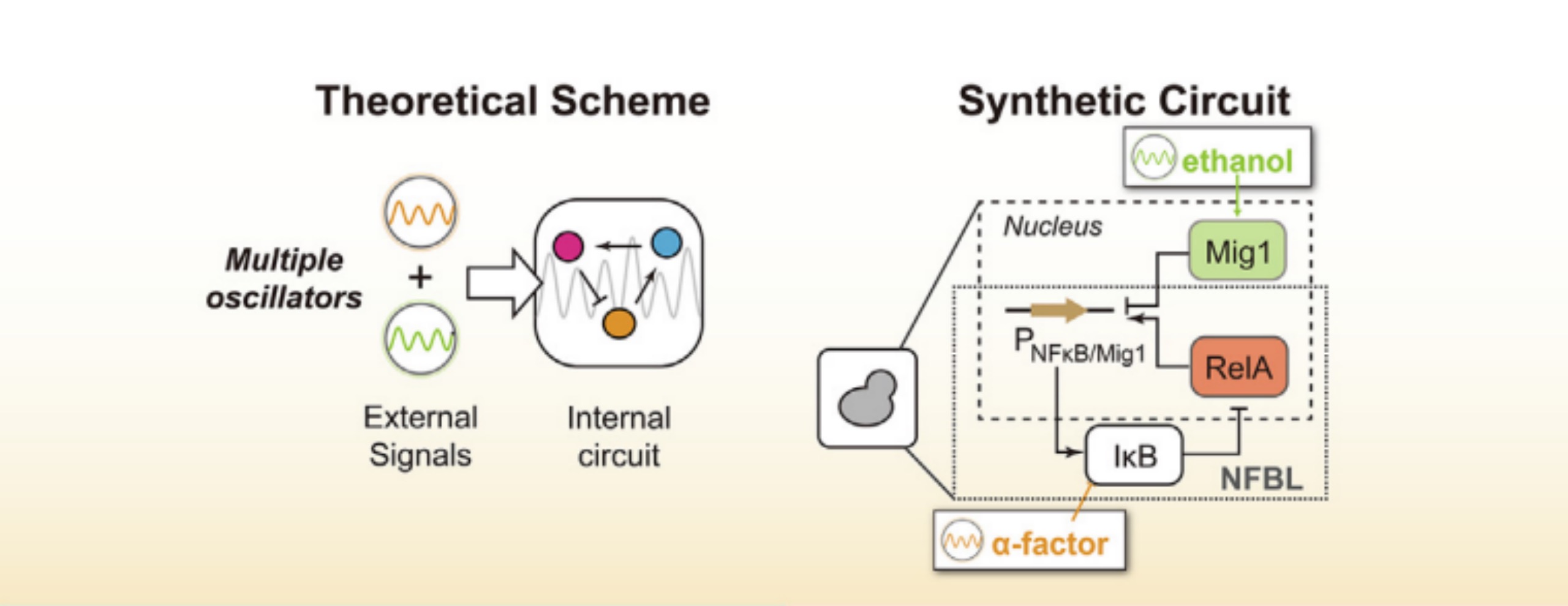
Figure 3 Basic design of dual input oscillation system
Based on this system and a mathematical model, the author found that the simultaneous presence of dual periodic signals could significantly expand the range of synchronization between internal and external oscillations, reduce the difference in cell population oscillatory dynamics, and increase the proportion of synchronized cells in the population. The model simulation results further showed that the dual input condition could delay the chaos. These results demonstrated that the effects of the two external periodic signals on the system were not simply linear superposition, but rather in a synergistic form.
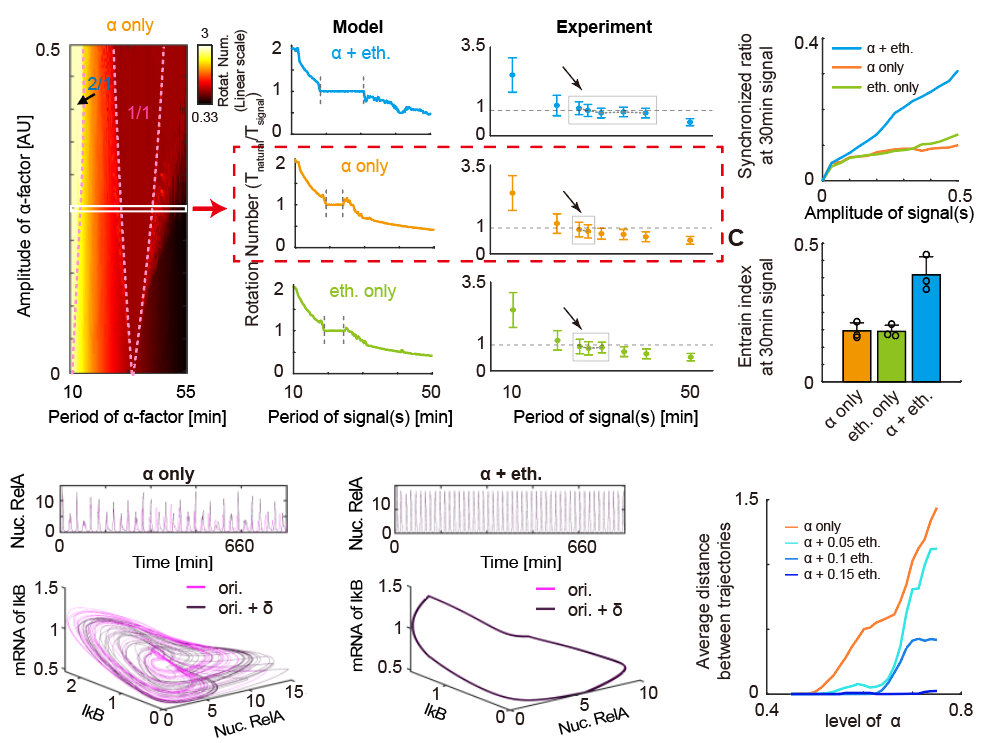
Figure 4 Expand the synchronization range, increase the proportion of synchronized cells, and delay the chaos with dual periodic signals
Furthermore, the author noted that adjusting the phase difference between the external signals could significantly change the amplitude of internal oscillations while keeping the period of internal oscillations unchanged, thus achieving theoretically strict “amplitude modulation without frequency modulation”. Further exploration indicated a high correlation between the signal phase combination that brought the maximum amplitude and the dynamic parameters of the internal system. In order to verify the universality of the above conclusions, the author also selected three different oscillation systems for numerical simulation. In Van der Pol oscillator, p53 oscillation system, and natural NF-κB oscillation system, the above conclusions were well replicated.
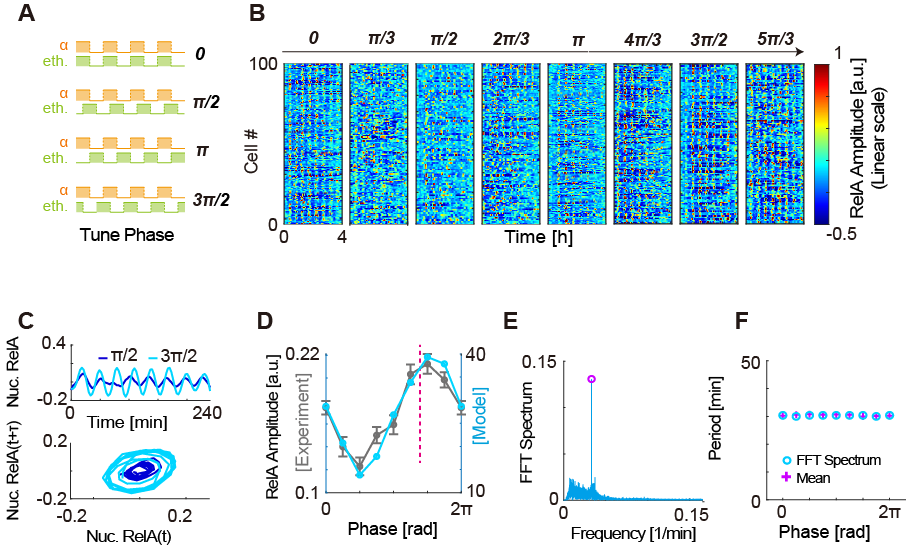
Figure 5 Adjust the phase of external periodic signals to realize amplitude modulation without frequency modulation
Finally, by introducing downstream fluorescent reporter genes, the author confirmed in experiments and models that the amplitude changes caused by this phase adjustment could indeed cause differences in gene expression levels. Such difference is independent of the baseline level and period of the oscillation.
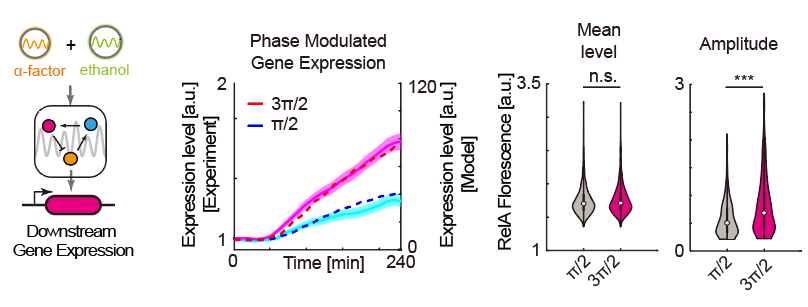
Figure 6 Regulate gene expression by changing the phase difference between external periodic signals
This study revealed the synergistic phenomenon in multi-coupled oscillation systems at both experimental and theoretical levels for the first time, discovered the unique phase regulation principle in such systems, and broadened the academic understanding of biological coupled oscillation systems and time regulation in biology. The dual input artificially synthetic oscillation system constructed in this study can provide a robust and reliable experimental platform for further exploring the basic problems in multi-coupled oscillation. This fundamental understanding of the design principle of living systems provides an important theoretical basis for artificial design of synthetic life and the understanding of the mechanism of complex diseases.
Dr. Mathias S. Helberg of the Bohr Institute of the University of Copenhagen (a visiting scholar of Shenzhen Institute of Advanced Technology, Chinese Academy of Sciences), doctoral candidate Jiang Yuanxu (a visiting student of Shenzhen Institute of Advanced Technology, Chinese Academy of Sciences), and postdoctoral fellow Fan Yingying (former PhD of the Quantitative Biology Center of Peking University) are the co-first authors of the paper. Researcher Wei Ping of Shenzhen Institute of Advanced Technology, Chinese Academy of Sciences, and Professor Mogens H. Jensen of the Bohr Institute of the University of Copenhagen are the co-corresponding authors of the paper. Academician Ouyang Qi and researcher Qian Long of the Quantitative Biology Center of Peking University provided valuable guidance and suggestions for this study. Lin Wei, a doctoral candidate of the Quantitative Biology Center of Peking University, and Zhang Zhibo, a former PhD of the Quantitative Biology Center of Peking University, made important contributions to the preliminary results of this study. This study was supported by the key Research and Development Program of the Ministry of Science and Technology, the National Natural Science Foundation, the Strategic Key Research Program of the Chinese Academy of Sciences, and Shenzhen Institute of Synthetic Biology.
References
1. Yoshioka-Kobayashi, K., Matsumiya, M., Niino, Y., Isomura, A., Kori, H., Miyawaki, A., & Kageyama, R. (2020). Coupling delay controls synchronized oscillation in the segmentation clock. Nature, 580, 119-123.
2. Mondragón-Palomino, O., Danino, T., Selimkhanov, J., Tsimring, L.S., & Hasty, J. (2011). Entrainment of a Population of Synthetic Genetic Oscillators. Science, 333, 1315 - 1319.
3. Zhang, Z., Wang, Q., Ke, Y., Liu, S., Ju, J., Lim, W.A., Tang, C., & Wei, P. (2017). Design of Tunable Oscillatory Dynamics in a Synthetic NF-κB Signaling Circuit. Cell systems, 5 5, 460-470.e5 .